1.3.6. Questions about Halo Problem¶

1.3.6.1. Differential Cross Section¶

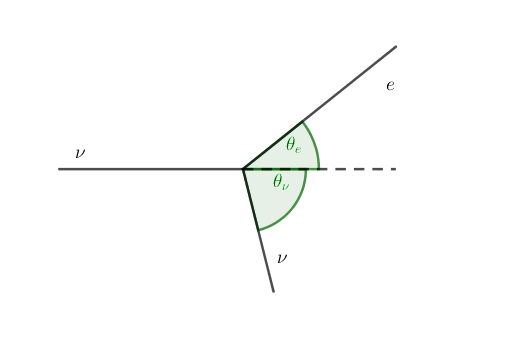
../../_images/neutrino-scattering.png — Fig. 1.188 Scattering of neutrinos off electrons.¶

Giunti derived the general form of differential cross section for all possible neutrino scatterings off electrons, equation 5.19 in his book [Giunti2007]. We plugin differential forms of Mandelstam variables to get the differential cross section related to neutrino scattering angles,

\[\frac{d\sigma}{d ( 2 E_\nu E_\nu' \cos \theta_\nu)} = \frac{G_F}{\pi} \left( g_1^2 + g_2^2 \left( 1 - \frac{E_\nu' \cos \theta_\nu}{m_e} \right) - g_1 g_2 \frac{E_\nu' \cos\theta_\nu}{ E_\nu } \right),\]

where \(E_\nu=p_\nu\) and \(E_\nu'=p_\nu'\) are the energies of neutrinos before and after scattering.

Applying conservation of four momentum, we have

\[p_\nu' = \frac{ m_e (m_e + p_\nu)}{ m_e + p_\nu - p_\nu \cos \theta_\nu }.\]

We are interested in the energy scale that \(p_\nu \gg m_e\). The energy of scattered neutrinos equations simplify to

\[p_\nu' = \frac{m_e}{ 1-\cos\theta_\nu }.\]

Plugin this into the differential cross section and keep only 0 orders of \(m_e/E_\nu\), we have

\[\frac{d\sigma}{d\cos \theta_\nu} = \frac{2 E_\nu m_e}{(1-\cos\theta_\nu)^2} \frac{G_F}{\pi} \left( g_1^2 + g_2^2 \frac{ 1 -2\cos\theta_\nu }{1 -\cos\theta_\nu} \right)\]

For neutral current, Giunti shows that the values of \(g_i\) are [Giunti2007]

\[\begin{split}g_1 &= g_2 = -0.27 \\ \bar g_1 &= \bar g_2 = 0.23,\end{split}\]

where bar indicates the values for antineutrinos.

Giunti’s formula

The differential cross section formula 5.29 in [Giunti2007] shows

\[\frac{d\sigma}{ d\cos \theta_e} = \sigma_0 \frac{ 4E_\nu^2 (m_e + E_\nu)^2 \cos \theta_e } { [ (m_e + E_\nu)^2 - E_\nu^2\cos^2\theta_e ]^2 } \left[ g_1^2 + g_2^2 \left( 1 - \frac{ 2 m_e E_\nu \cos^2\theta_e }{ (m_e+E_\nu)^2 - E_\nu^2 \cos^2\theta_e } \right)^2 - g_1 g_2 \frac{ 2 m_e^2 \cos^2\theta_e }{ ( m_e + E_\nu )^2 - E_\nu^2 \cos^2\theta_e } \right].\]

We are interested in supnernova neutrinos whose energy is usually larger than mass of electrons. We set \(m_e/E_\nu \to 0\).

\[\frac{d\sigma}{ d\cos \theta_e} = \sigma_0 \frac{ 4 E_\nu^2 \cos \theta_e } { [ 1 - \cos^2\theta_e ]^2 } .\]

We need a relation between \(\theta_e\) and \(\theta_\nu\). The way to derive it is to use conservation of four momentum.

\[\begin{split}E_\nu' + \sqrt{p_e'^2 - m_e^2} &= m_e + E_\nu \\ p_\nu' \sin\theta_\nu + p_e' \sin\theta_e &= 0\\ p_\nu' \cos\theta_\nu + p_e' \cos\theta_e &= p_\nu.\end{split}\]

We imediately notice that for backward scattering, \(\theta_e = \pi + \theta_\nu\).

Giunti2007(1,2,3): Guinti & Kim, Fundamentals of Neutrinos and Astrophysics

1.3.6.2. MISC¶

Reflection coefficients for neutrinos and anti-neutrinos are different.