1.5.1.3. 2017-10 Group Meeting¶
1.5.1.3.1. 2017-10-05¶
Bipolar model with matter effect. (link)
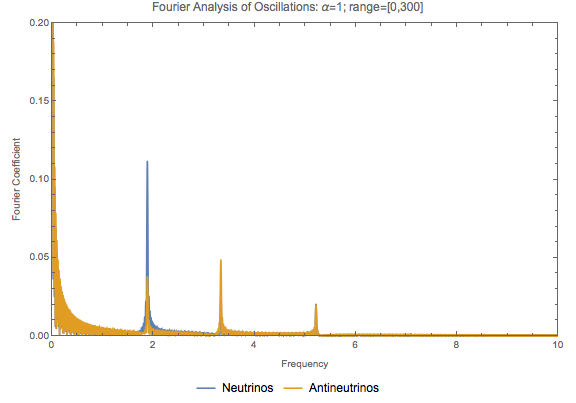
Fig. 1.211 With \(\alpha=1\), \(\lambda=\cos 2\theta_v\), \(\mu=3\omega\), where everything is in unit of \(\omega\). Range of calculation is \([0,300]\).¶
The frequencies are 1.95547, 3.39437, 5.25602.
The plots obviously show that the NFIS’s going through three fourier modes,
Guess
It should be
Before we work out the data fitting, it would be nice if I can find the relation between the parameters and coefficients and frequencies.
I should work out \(\alpha=0.5,0.8,1,1.2,1.5\), \(\mu=0.5,1,2,3\), as well as some change in \(\lambda\).
Observations
Generally speaking, small \(\alpha\) enhances the first frequency.
Frist we calculate \(\lambda=\cos 2\theta_v\) and \(\mu=3\)
For \(\alpha=1\)
frequencies: {1.95547, 3.39437, 5.25602}
A: {0.111267, 0.0282127, 0.021448}
Abar: {0.0397217, 0.0496267, 0.0205751}
For \(\alpha=0.8\)
frequencies: {1.50333, 3.4475, 4.96213}
A: {0.146606, 0.0257919, 0.0199095}
Abar: {0.0434389, 0.038914, 0.0167421}
For \(\alpha=0.6\)
{1.16424, 3.59444, 4.72477}
{0.189593, 0.021267, 0.0167421}
{0.0459276, 0.0316742, 0.0153846}
For \(\alpha=0.5\)
frequencies: {1.03651, 3.58314, 4.6279}
A: {0.225792, 0.0217194, 0.0134389}
Abar: {0.0459276, 0.0255656, 0.0104072}
So the amplitude for neutrinos seems to be changing linearly as a function of \(\alpha\).
Keep \(\alpha=1\) and \(\lambda=\cos 2\theta_v\), while change \(\mu\).
For \(\mu=2\).
Frequencies: {1.46942, 2.91624, 4.38567}
A: {0.122172, 0.0303167, 0.0217195}
Abar: {0.0312217, 0.0533937, 0.0190045}
I consider the amplitudes unchanged but the frequencies are shifted to lower values.